US COVID19

March 30th, 2020.
Fabio Gori, University of Rome Tor Vergata, Rome, Italy.
The update of today is represented by the following figure with the asymptotic value above 250,000.

 

Today I am presenting also the same figure per 1000 capita. The ratio is around 0.8.

 

March 28th, 2020.
The update of today is represented by the following figure with the asymptotic value of above 200,000.

It is presented also the ratio with 1000 inhabitants of the population.








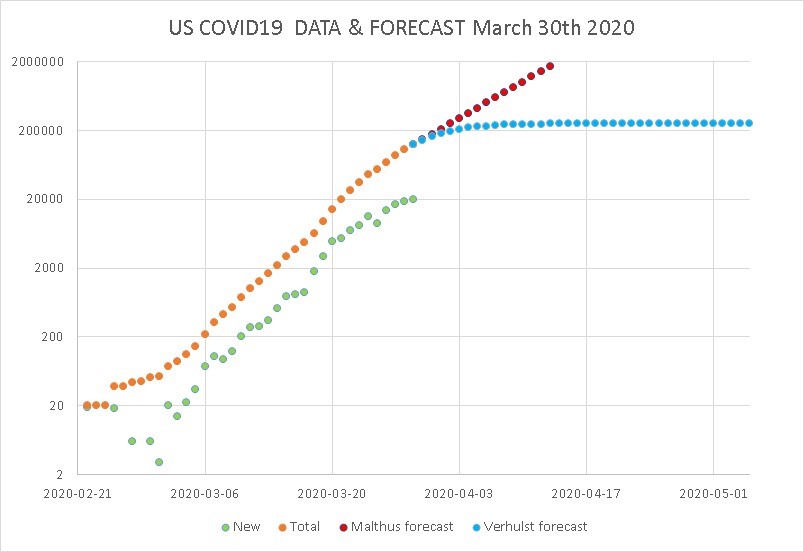
March 27th, 2020.

The update of today is represented by the following figure with the asymptotic value of about 180,000.



March 25th, 2020.

Today I have studied the United States COVID19 cases with the data up to yesterday, March 24th, 2020.
The first figure is relative to the new and total cases, reported in a linear scale. It is quite evident that the exponential curve of the total cases is very steep.


The second figure reports the same data in a logaritmic scale where the exponential curve become a linear increase.

In  the following figure the forecasts obtained with the Malthus and Verhulst approaches are reported in a linear scale. The finale asymptotic value is above 160,000 cases.

The same results are reported in a logaritmic scale, which evidences also the linear increase of the new cases.

In the next days I will update the forecast with the new data.

Commenti

Posta un commento

Post popolari in questo blog

COVID19 DATA AND FORECAST IN EU AND US, UPDATE OF APRIL 5th, 2020.

Immagine
April 5th, 2020. Fabio Gori, University of Rome Tor Vergata, Rome, Italy. www.termofluidodinamica.it   eucovid19.blogspot.com usacovid19.blogspot.com covid19forecast.blogspot.com   forbigaio.blogspot.com Today I have assembled data and forecast of the April 3rd, 2020, of the COVID 19, presenting the total values  of EU, US, Italy, France, Germany, Spain, and United Kingdom for the first time, as well as the data and forecast for 1000 capita. The forecast of the new cases for Italy has been posted in this blog, with the trend to decrease during the month of April, but I have reported it also here. EU 27. The forecast of the total cases is toward over 760,000, while the same data, rated per 1000 capita, is 1.7. United States. The forecast of the total cases is toward over 460,000, while the same data, rated per 1000 capita, is 1.4. Italy. The forecast of the total cases is toward over 110,000, while the same data, rated per 1000 capita, is 1
Continua a leggere

COVID19 DATA AND FORECAST IN EU AND US, UPDATE OF APRIL 7th, 2020.

Immagine
April 7 th, 2020. Fabio Gori, University of Rome Tor Vergata, Rome, Italy. www.termofluidodinamica.it   covid19forecast.blogspot.com eucovid19.blogspot.com usacovid19.blogspot.com   forbigaio.blogspot.com Today I have assembled data and forecast of the April 6 th 2020 of the COVID 19, presenting the  forecast of the total and new cases in Italy and EU, the total ones in USA, as well as data and forecast for 1000 capita. Italy. The forecast of the total cases  is over 108,000 and the same data rated per 1000 capita is 1.8. Today I present for the third day the decrease of the new cases in Italy, showing the same forecast done on April 4th, but where I have put also the new data of the 5th and 6th of April. As is shown by the figure the forecast seems to be still valid since the real data presents oscillations around the Verhulst forecast, which is a mean one.   EU. The forecast of the total infections is somewhat above 700,000, while the same data, rated per
Continua a leggere

COVID19 FORECAST OF THE DECREASE OF NEW CASES IN CHINA AND ITALY

Immagine
April 5th, 2020. Fabio Gori, University of Rome Tor Vergata, Rome, Italy. www.termofluidodinamica.it   covid19forecast.blogspot.com   forbigaio.blogspot.com usacovid19.blogspot.com eucovid19.blogspot.com Today I have forecasted the increase and decrease of the new cases of COVID19 applying the equation of Malthus and Verhulst to China and Italy. As far as China is concerned, the increase of the new cases follows the exponential increase of Malthus, but, after the maximum, the Verhulst equation is required, which appears in reasonable agreement to the real data happened in China. The same procedure has been applied to the new cases in Italy showing a trend in agreement to the real data available up to today, during the increase and the decrease. The increase follows the Malthus equation up to the maximum, while the decrease requires the Verhulst equation. From the present forecast it is possible to expect a small number of new cases by the end of this month of April.
Continua a leggere